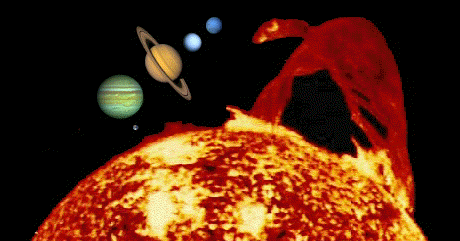
The Sun and the 5 largest planets at a scale of 3200 km/pixel

Build Your Own Scale Model of the Solar System from Scratch!

An activity by Padi Boyd
related to the song:
'Nine Planets'

Note: This song was written when Pluto was still considered a planet. We leave Pluto in this activity because we still love Pluto.

The object of this exercise is to give participants a sense of the distances in the solar system. The sizes of the planets, and their distances, have all been scaled by the same amount in this activity. So if you think of the Earth as being the size of a chickpea, the Moon is the size of a small tapioca pearl, located 20 cm away. This is also an excellent way to introduce or reinforce the concept of scale. Challenging the students to discover and use various scale factors throughout also gives them experience with the metric system, keeping track of units, and the algebra involved in determining a scale factor from the data given.

What is a Globe, Really?

Introduction: A natural place to start is with the Earth globe in your classroom. Engage students in a discussion about an Earth globe. What information does it preserve about our planet? Does it accurately portray the locations of the continents and the oceans, their sizes with respect to one another? Does it accurately represent the portion of the surface of the Earth that is covered with water? Yes it does. And the reason it does is because it is actually a scale model of the planet. Depending on the level of your students, you could do the following exercise:

Measure the circumference of the globe in centimeters, and from this calculate the diameter of your globe. The Earth is 12,740 km in diameter. How much bigger is the actual Earth than the globe? This number is the "scale factor".

		C = globe circumference (in cm) = _____________ cm
		C/pi = globe diameter D   = ____________________ cm

D cm = 12,740 km so:


		1 cm = 12, 740/D km = 12, 740/______________ km
		1 cm	= __________________________________ km

This is your scale factor.

Everything on your classroom globe would have to be multiplied by (or scaled by) this number to be the same size as it is on Earth. The same number scales all the oceans and continents on the globe. The Moon's diameter is about 3500 km. Using the same scale factor, have students discuss what common object could represent the Moon. Moon (cm) = Moon (km) / scale factor

		= 3500 km / ____________ cm
            	= ______________________ cm

A softball is usually about right if you're using a standard size globe. The distance of the Moon from the Earth is 400,000 km. Using the same scale factor, ask one student to take a softball the appropriate distance away from the globe. Depending on the size of your room, your Moon student may need to go out in the hallway! Moon's distance(cm) = Moon's distance (km) / scale factor


		= 400,000 km / _____________ cm
		= __________________________ cm

We're going to use the same idea of a scale model for the entire solar system. But in order to fit the entire solar system into a reasonable distance, we'll need a different scale factor, one in which the Earth will be much smaller than the globe. With the new scale factor, we'll be able to walk most, if not all of the solar system, in a class period. The new scale factor is:

Solar system tour scale factor: 1 mm = 2000 km.

Table: Solar System Objects and their scaled diameters (D) and distances (d) from the Sun

Object	D (km)	D (mm)	d (km)	d (m)
Sun	1,391,900	696	---	---
Mercury	4866	2.4	57,950,000	29
Venus	12,106	6.	108,110,000	54
Earth	12,742	6.5	149,570,000	75
Mars	6760	3.3	227,840,000	114
Jupiter	139,516	70	778,140,000	389
Saturn	116,438	58	1,427,000,000	714
Uranus	46,940	23	2,870,300,000	1435
Neptune	45,432	23	4,499,900,000	2250
Pluto	2274	1.1	5,913,000,000	2957

In the Table above, D is diameter of the object. The distance of the object from the Sun is d, and is given in meters. This is also the object's orbital radius about the Sun.

Prospecting for planets

Offer your students tape measures and numerous spherical objects, all of which can be obtained at low cost at a supermarket. Include: grapefruit, orange, tangerine, cherry tomatoes, pepper corns, peas, coriander seeds, nonpareil sugar topping, tapioca pearls, chick peas, and anything else that catches your eye at similar, as well as intermediate, sizes. Have the students use the data table above to explore this collection of objects in search of representatives of the planets. Try to get as close as possible to the diameters in mm listed in the table for each solar system object, excluding the Sun.

Our group used: pepper corn (Mercury), chickpea (Venus), chickpea (Earth), green pea (Mars), large orange (Jupiter), plum (Saturn), cherry tomato (Uranus), cherry tomato (Neptune), coriander seed (Pluto).

Assign one or two students to each object in the solar system. Have them become your local experts on that object. During your solar system walking tour, ask your experts to share their knowledge about their object.

Ready for the Tour!

In order to take your solar system walking tour, you must first get an estimate of how many steps it takes to walk one meter. A typical group of 15-year-olds will walk an average of two steps per meter. So multiply d, in meters, by 2, and walk that number of paces to get to the distances from the Sun. So, with a large object, such as a beach ball, as the Sun at your starting point, walk approximately 58 steps and place the object representing Mercury. Look back at the Sun to see how large it looks, and how far away it is. Hear your experts' report on Mercury. Now move on 50 more paces (54-29) x 2. Place the object representing Venus here. Listen to the Venus experts' report. Notice the size of the Sun from this distance, and the distance you are from Mercury. Continue walking on, and placing your planet objects at the locations indicated in the table above. Depending on how much time you have, you may wish to cut your walk short once you arrive at Jupiter, and just quote the distances to the outer planets, estimating how far you'd have to walk to get there.

Students should walk away from the solar system tour with a new appreciation for the enormous distances between objects in the solar system, as well as their relative sizes.

Extensions

You can include the Moon in the scale model of the solar system.

At this scale it would be about 2mm across, and 20 cm from the Earth. Likewise, you can include Jupiter's larger moons, the Galilean satellites. Have your Jupiter team research their sizes and distances from Jupiter, and then scale these appropriately.

You can sprinkle grains of salt or sand at 210 meters to represent the asteroid belt, which lies between Mars and Jupiter. But sprinkle very lightly -- the average distance between the larger asteroids is more than ten times the Earth/Moon distance! That's two meters or more on our scale.

You can include Kuiper Belt objects, which are rapidly being discovered near and beyond the orbit of Pluto. Use objects about half the diameter of Pluto and smaller, scattered at roughly the same distance from Neptune, and beyond.

Reference

This lesson was motivated by a similar solar system model which can be found at http://members.aol.com/ARIELSTIN/page4.html. Major changes are adjusting scale factors so that only the metric system is used, and adjusting the method so that it matched that which was field tested with participants of the North Bay Science Project educator workshops held at Sonoma State University in Rohnert Park, CA in July 2001.